Table of Contents
Slab :
Slab is a common structural element consisting of a flat, horizontal surface with depth much smaller than width and span. The frequent use of slab is found as floor and roof in building, decks in bridges, top and bottom of tanks, road pavement and so on. The two way and one way slab design are mainly focused.
The thickness of steel reinforced slab typically between 100 and 500 mm thick. In building, the slab thickness is normally between 100 to 200 mm. It is advisable not to use slab of thickness greater than 150 mm in building because it increases the self-weight which causes severity in earthquake.
A slab supports and transmit load, to supports primarily by flexural, shear and torsion, to wall, beam or directly to column.
Slab Design:
A slab is generally designed as flexural element considering 1 m wide strip. Although slab is not casted in 1 m strip, it gives satisfactory design result on using 1 m strip. The reason for designing only for flexural member is that the shear stress are not critical and reinforcement provided for flexural is enough to resist. But it may be necessary in one way slab only if span is small and load is large.
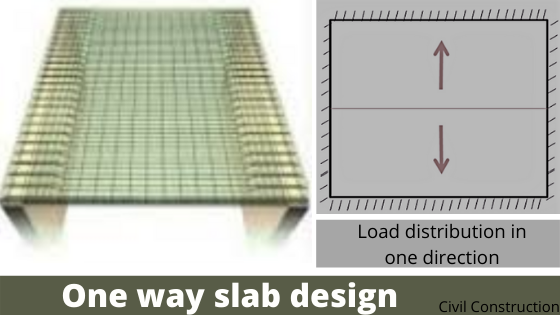
One-way slab:
One way slab is the slab that is supported on two parallel sides carrying loads by flexural in the direction perpendicular to the support. The slab supported on four sided with a ratio of longer to shorter span greater than 2 are also called as one way slab.
Since load is distributed in only one direction, reinforcement is also needed in only one direction which is called main reinforcement. Steel reinforcement at the right angle to main reinforcement which is called distribution reinforcement. The distribution reinforcement is provided despite it does not contribute on load transfer. It helps to distribute superimposed load properly and also helps in distributing secondary stresses due to temperature change, moisture variation, shrinkage, and so on.
Since most of the load is carried on shorter span, main reinforcement is only to be designed for only one direction (shorter span). The distribution reinforcement should be provided 0.12% of bD which is minimum.
Simply supported:
The maximum bending moment is at mid-span equal to wl²/8
The maximum shear force is at support equal to wl/2
The maximum deflection is at mid-span equal to 5wl⁴/ 384EI
Fixed slab
The sagging moment at the mid-span is wl²/24 and hogging moment at support is wl²/12.
The maximum shear force is at support, equal to wl/2
The maximum deflection is at mid-span, equal to wl⁴/ 3.84EI
Cantilever slab
The maximum bending moment is at mid-span, equal to wl²/2
The maximum shear force is at support, equal to wl
The maximum deflection is at mid-span, equal to wl⁴/ 8EI
Continuous slab
The precise determination of the theoretical bending moments involve mathematical labor. And due to simplified assumption, the theoretical bending moment being greater than those actually realized. Hence approximate moment coefficient recommended in IS 456, which was derived through idealization done using a series of long strip, should be used to calculate moment and shear force.
Before going to design step it would be better if you know about the ‘Point to be known before design’ which you can found in preliminary design of slab.
One-way slab design steps:
Step 1. Calculate aspect ratio and determine either it is one-way or two-way slab.
Aspect ratio = longer span / shorter span
If greater than equal to 2, it is one-way slab and if less, it is two-way slab.
Step 2. Determine the minimum thickness of slab from serviceability criteria of deflection control.
As it is already discussed in preliminary design of slab, CLICK HERE to reach there.
Step 3. Calculate the effective span of slab from IS 456 clause 22.2 which is least of following values
Center to center of supports or clear span + width of support
Clear span + effective depth of slab
Step 4. Determine the total factored load due to live and dead load considering 1m wide strip.
Dead load is calculated from IS 875 part 1 and it mainly includes self-weight, floor finish and partition load whereas live load is calculated from IS 875 part 2.
Step 5. Calculate the factored design moment Mu and factored design shear force Vu.
It’s formula is already mentioned above for different conditions (simply supported, continuous,…)
Step 6. Check the thickness of slab.
The thickness of slab is calculated for given value of factored moment then check with initial value. The thickness calculated from factored design moment should be less than initial value if not, revise with greater depth.
Mu = Mu,lim = 0.36 fck b xu [d – 0.42 xu]
Step 7. Determine the area and spacing of main reinforcement.
The area can be calculated from given formula;
Mu = 0.87 fy As [ d – fy As/ fck b]
The spacing of main reinforcement bar should not be more than 3 times the effective depth of solid slabs or 300 mm whichever is smaller.
And the minimum area of main steel reinforcement is equal to 0.12% of bD.
Step 8. Check the adequacy of depth from deflection control criteria.
The thickness initially obtained is through different assumption and after calculating area of reinforcement provided, it is easier to calculate exact modification value. (IS 456:2000 clause 23.2.1)
The calculated ratio of shorter span to depth should be greater than initially obtained from assumed value.
Step 9. Check for development length
From IS 456:2000 clause 26.2.1
The development length is given by
Ld = 0.87 fy Փ / 4Ⴀbd
Step 10. Check for shear
For one-way slab, it would not be a bad practice to check for shear.
From IS 456:2000 clause 40.2,
The design shear strength Ⴀuv shall be less than equal to shear strength of concrete kႠuc
Step 11. Calculate the distribution reinforcement.
From IS 456:2000 clause 26.5.2.1; the distribution reinforcement should be provided 0.12% of b D if high strength deformed bar or 50% of main reinforcement whichever is greater.
The spacing of distribution bar should not be greater than 5 times the effective thickness of slab or 450mm whichever is smaller.
Step 12. After designing all, all reinforcement drawing should be drawn.
ALSO READ