Table of Contents
Primary beam supporting secondary beam:
Primary beam supporting secondary beam means that the secondary beam is resting on the primary beam. Do you know what is primary beam and secondary? Hope you have view my previous post. If not, click here for primary beam and for secondary beam click here. As it is already mentioned in secondary beam that it is treated as simply-supported despite it is casted monolithically. Since simply supported, there is no moment developed at the joint and no torsion developed. But in reality, there is development of torsion in primary beam due to secondary and this can be simply solved by the special arrangement of reinforcement. In spite of assuming as simply supported, it gives satisfactory result for the design and analysis.
The secondary beam only transfer loads to primary beam so during calculation, we should take point load at the junction of these two types of beam. Thus, primary beam experiences both uniformly distributed load and concentrated load if it support secondary beam.
Design of primary beam supporting secondary beam:
The design of primary beam with secondary beam is almost same as normal beam supporting on column in both ends. The only difference is concentrated load at the intersection point for the calculation of moment. But the arrangement of reinforcement is different. Are you thinking what that special reinforcement arrangement is? Just wait, you will know it after completing the whole content. Let’s understand all about the primary beam supporting secondary beam from an example.
Numerical:
Design a primary beam supporting secondary beam of the given institutional building plan which is aligned in x-axis supporting the secondary beam at the mid-span. Grade of concrete = M30 and grade of steel = 500D. Slab thickness = 130 mm and Partition material= AAC block of thickness 150 mm having unit weight 8 KN/m3. Live load = 3 KN/m3 and floor finish = 1.5 KN/m3. Assume other data if necessary.
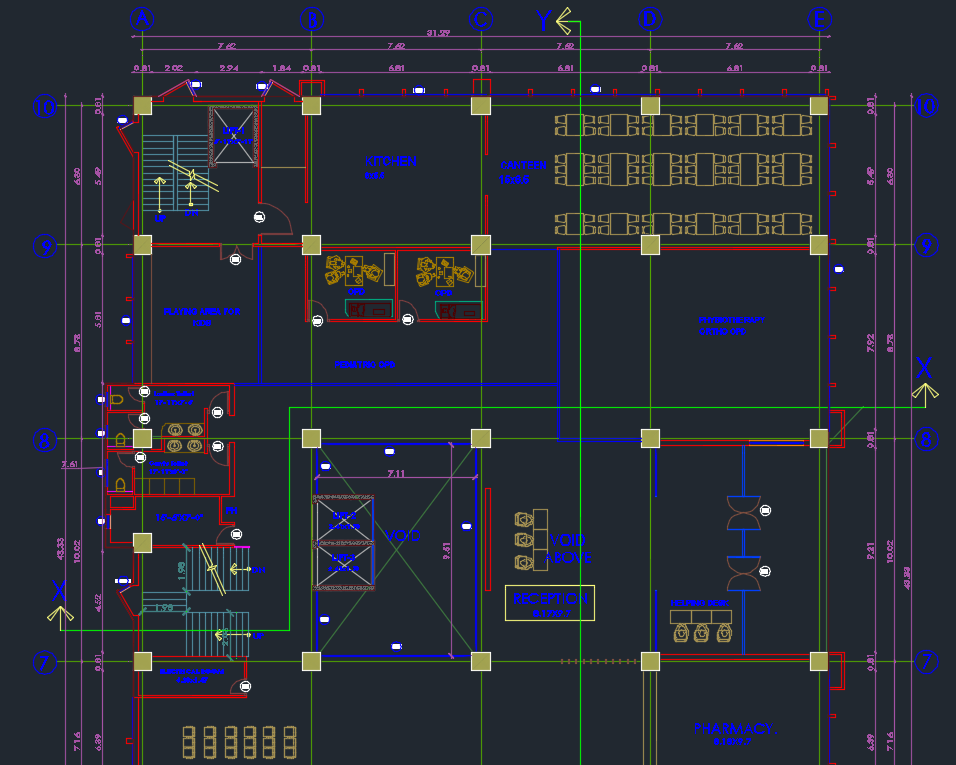
The SAP modeling plan is also attached below in which the light green thick line is secondary beam and the pink one is primary beam. We can see that the primary beams along x-axis are supporting the secondary beams.
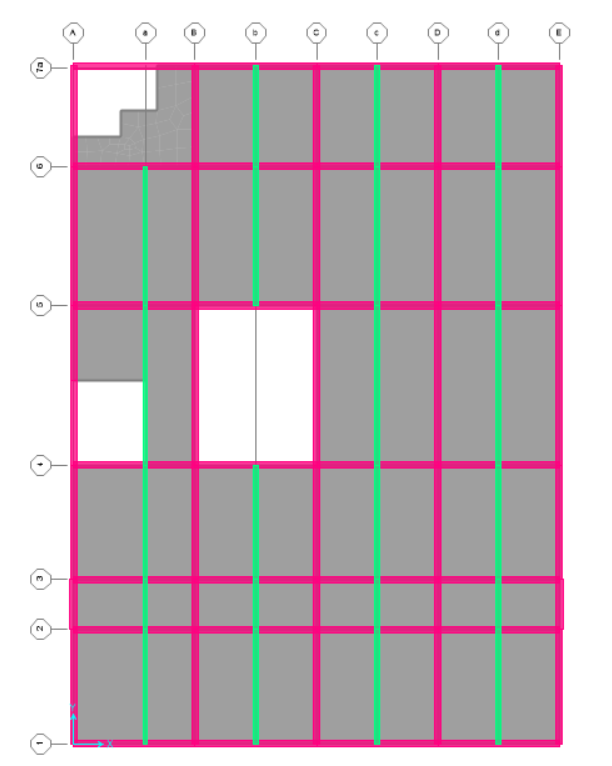
For the design of beam along x-axis, we have to choose the critical beams. Since all the beam have equal span, we can’t decide from this which one to choose. But the critical one is that which have to support greater load and for this the beam which have wider slab should be consider. For this, we know, the beams of row 8 shown in architectural plan are critical. The reason is that it have to resist half load from slab of both side (10.02 m and 8.73 m)
- Preliminary design of beam
The detail about the preliminary design is discussed before. If you want to go there, then click here.
The preliminary design is based on the serviceability condition of deflection criteria. From IS 456:2000 clause 23.2.1,
L/d ≤ αβγδλ
Assume d = l/20.7 [For continuous beam and Fe 500D ]
Where l is effective span which can be calculated from clause 22.2 of IS 456:2000.
So, d = 7.62/20.7 = 0.368 m
Assume diameter of bar = 20 mm and clear cover = 25 mm
Since it has to resist secondary beam also and for the construction simplicity,
Adopt same size as others beam designed before.
Adopt overall depth = 600 mm
So effective depth = overall depth – clear cover – half of diameter of bar
= 600 – 25 – 20/2 = 565 mm
And width = 400 mm
2. Load calculation
- Partition load
Thickness of wall = 150 mm
Height of wall = floor to floor height – depth of beam
= 4.27 – 0.6 = 3.67m
Unit weight of partition material = 8 kN/m3 [ since AAC block as partition, if brick-wall then 19.2 kN/m3]
So, weight of partition wall = 8 * 3.67 * 0.15 = 4.4 KN/m
- Self-weight of beam
Size of beam = 250mm * 600 mm
Unit weight of concrete = 25 kN/m3
So, self-weight of beam = 25 * 0.4 * 0.6 = 6 kN/m
- Load from secondary beam:
Load from slab
Since the slab are one-way, the secondary and primary beam along y-axis takes all the loads. [From detailed design of slab, to learn more click here.]
Thickness of slab = 130 mm
Live load = 3 kN/m3
Floor finish = 1.5 kN/m3
So, weight of slab = unit weight * thikness of slab
= 25 * 0.13
= 3.25 kN/m2
Self-weight of beam
Size of beam = 400mm * 600 mm
Unit weight of concrete = 25 kN/m3
So, self-weight of beam = 25 * 0.4 * 0.6 = 6 kN/m
Length of the secondary beam loaded on primary beam = half the effective distance in left and half in right
= 10.02/2 + 8.73/2
= 9.375 m
Width of the slab loaded on secondary beam = half the effective distance in left and half in right [since one way slab]
= (3.82+3.82)/2
= 3.82 m
So, concentrated load from secondary beam = (live load + floor finish + slab weight + beam load) * loaded width
= [(3 + 1.5 + 3.25) * 3.82 + 6] * 9.375
= 333.8 KN
Factored concentrated load P = 1.5 *333.8
= 500.7 KN
Factored UDL w = 1.5 * (partition load + beam weight)
= 1.5 * (4.4 + 6) = 15.6 KN/m
3. Moment calculation
In the previous post of beam, we had use SAP2000 data for the design of moment. But here along with the data of SAP, we also try to calculate from code.
From IS 456:2000 code table 19, we get
Maximum moment is at the support next to end support, that is
For UDL, – 1/10 for live load and -1/9 for dead. AND all UDL load is dead.
For point load, using formula as fixed end moment.
So , Mu = PL/ 8 + w L2/ 9
= 500.7 * 7.62 / 8 + 15.6 * 7.622/9
= 577.56 KN-m
From structural design analysis software SAP2000, [ you can use any other software also like ETABS and STAAD PRO]
In beam, we have to calculate reinforcement in support and mid span.
At support,
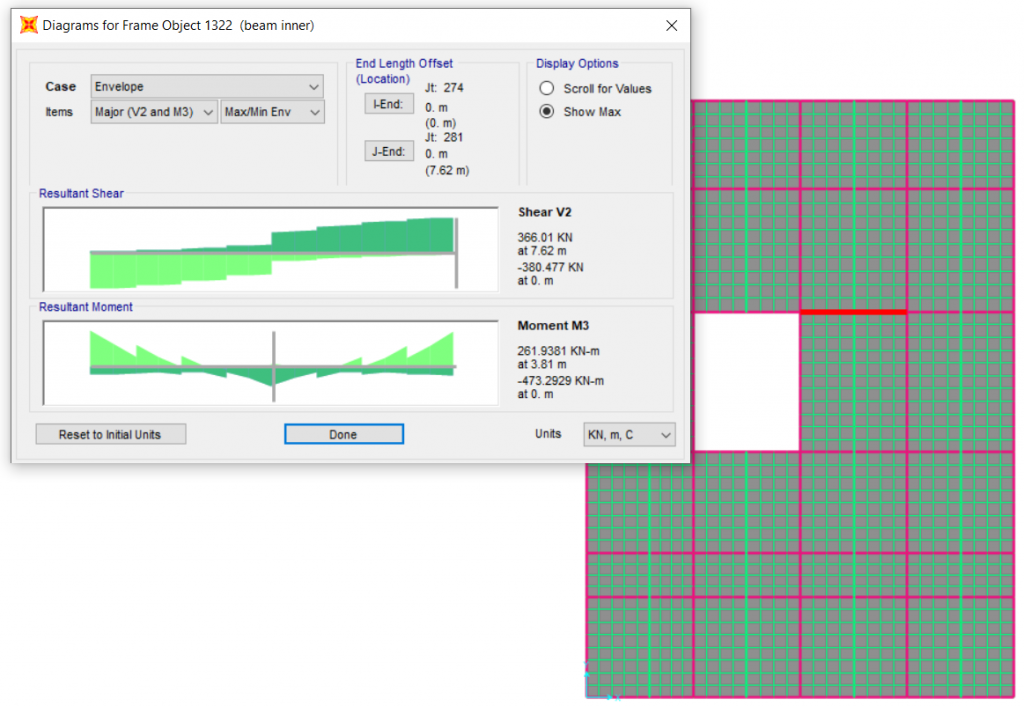
From SAP2000, [figure above]
Max. Moment at support = – 473.3KN-m
Shear force at support = 380.5 kN
At mid-span:
Since the curtailment or bent-up begin from the point where the bending moment is minimum or zero. For this case, the maximum moment from fig. above
Moment for mid span = 87.24 KN-m
Comparison: why the data obtained from code and structural design software differs?
From the above result, we can see that the maximum moment at the support differs by 105 KN-m. This is due to 1) Assumption of fixed end for moment calculation from point load. 2) The span length of all beam is same but load on each beam differs because there is no slab and secondary beam in one side of primary beam which makes error in the result from code.
So let’s take the data from SAP 2000 for further analysis.
4. Check for singly or doubly reinforced beam
For this, we have to calculate limiting moment
Mu,lim = 0.133 fck b d2 [ for fe 500]
= 0.133 * 30 * 400 * 5652 [fck = 30 N/mm2]
= 509.48 KN-m
So singly reinforced beam
5. Calculation of tensile and compression reinforcement.
For singly reinforced beam
At support,
Mu = 0.87 fy Ast [ d – fy Ast / fck b ]
473.8 * 106 = 0.87 * 500 * Ast [565 – 500 * Ast/ 30 * 400]
Ast = 2327.17 mm2
At mid-span, [ since the moment for top and bottom bar is nearly same so calculate for only one]
Mu = 0.87 fy Ast [ d – fy Ast / fck b ]
87.27 * 106 = 0.87 * 500 * Ast [565 – 500 * Ast/ 30 * 400]
Ast = 365 mm2
6. Calculation of number of bar provided and check for min. and max. limits
The assumed diameter of bar = 20 mm
Area of a bar = 3.14 * 202/4
= 314 mm2
Checking limits:
- the maximum area of reinforcement provided = 4% of bD = 9600 mm2
- the minimum area of reinforcement required = 0.85 * bd/fy = 384.2 mm2
At support,
Area of top [tensile] bar = 2327.17/314 = 7.4 nos
At mid span,
Area of bottom [tensile] bar = 384.2 / 314 = 1.22 nos
satisfying the limit so,
provide two bottom bar of diameter 20 mm throughout
provide two top bar of dimeter 20 mm throughout
for extra top bar a support = 8-2 = 6 nos
7. Arrangement of bar with spacing check
From code IS 456:2000 table 15, the clear distance between the bars should not be greater than 150 mm for Fe 500 with no moment redistribution.
Provide 3/3 number of 20mm bar on both top and bottom layer of beam at midspan.
Checking spacing = (width – 2 * clear cover – nos * diameter of bar ) / ( nos of bar -1)
= (400 – 2 * 50 – 3 * 20) / 2 = 120mm ok
Spacing of 7 bar in top support = (width – 2 * clear cover – nos * diameter of bar ) / ( nos of bar -1)
= (400 – 2 * 25 – 8 * 20 ) / ( 8-1 ) = 27.14 mm ok
8. Check for deflection
For deflection control criteria, using IS 456:2000 clause 23.2.1
For modification factor for tension reinforcement,
fs = 0.58 fy * area required / area provided
= 0.58 * 500 * 384.2/942.47
= 120
Percentage of steel = 3*314.16 *100 / 400*600 = 0.39 %
From fig.4, modification factor for tension reinforcement = 2
From fig. 5, for compression reinforcement 0.39% , modification factor = 1.1
From fig. 6 modification value = 1
Allowable l/d ratio = basic value * modification value
= 26 * 2 * 1.1 *1 = 57.2 > Assumed (20.7)
9. Check for shear
First calculate nominal shear stress Ⴀv = Vu / bd
= 380.5 * 1000 / 400 * 565
= 1.69 N/mm2
From table 19 of code IS 456:2000, calculate design shear stress Ⴀc
For steel percent 0.39% and M30 grade of concrete,
Ⴀc = 0.37 +(0.50-0.37)*(0.39-0.25)/(0.5-0.25)
= 0.44 N/mm2
and from table 20, the maximum shear stress Ⴀc,max = 3.5 N/mm2
If Ⴀv < Ⴀc < Ⴀc,max, shear reinforcement are to be designed.
10. Calculation of transverse reinforcement with spacing
The shear reinforcement can be calculated from the code IS 456:2000 of clause 40.4 (page 73).
Near the support, there is only stirrups to resist the shear force.
Shear resisted by reinforcement = Total shear minus shear resisted by concrete.
= (1.69 – 0.44) * 400 * 565
= 282.5 KN
Now,
Assume diameter of stirrups = 8 mm
Since two-legged stirrups so,
Area = 2 * 3.14 * 82/ 4
= 100.5 mm2
So,
Vus = 0.87 fy Asv d / sv
282500 = 0.87 * 500 * 100.5 * 565 / sv
Sv = 87.45 mm
At the point of bar bent-up
There are bent-up bars to resist shear stress beside the traverse reinforcement. The contribution of bent-up bar can’t be taken more than 50% of total shear resisted by reinforcement.
Since we have not provided any bent-up so all the shear is resisted by the vertical stirrups only. And the shear force decrease as we moves from support to mid-span so we can increase the spacing of stirrups.
The spacing of transverse reinforcement should not be greater than 300 mm or 0.75d .
0.75 d = 0.75 * 565 = 423.75
So provide stirrups of 8 mm diameter @ 80 mm c/c at support
And stirrups of 8 mm diameter @ 100 mm c/c at mid-span.
From the whole design procedure, it shows that the beam of size 400 mm * 600 mm gives satisfactory result. And also for economic design you can go for next trial with smaller dimension and check out all procedure.
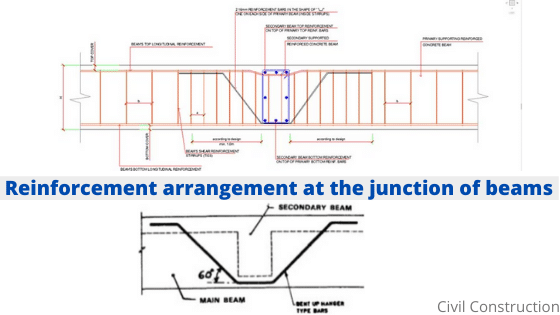
The arrangement of reinforcement at the junction of primary beam supporting secondary beam is shown as :
ALSO READ

