Raft foundation design

Example of Raft Foundation design:

The higher structural loads and low bearing capacity of soil demands for raft foundation design. The example of raft foundation design is discussed here in details.

Example: Design a raft foundation of a raft foundation shown below. Some of the design constrains required for raft foundation design are as,

Design Constants:

Unit weight of soil (γ) = 18 KN/m3

Service Load (P) = 188190.67 kN

Service load includes the total axial forces applied from column, lift wall weight , and load from basement walls.

Grade of Concrete = M30

Grade of steel = Fe 500D

Bearing Capacity (q) = 150 KN/m2

Angle of Repose of soil (Ø) = 30⁰

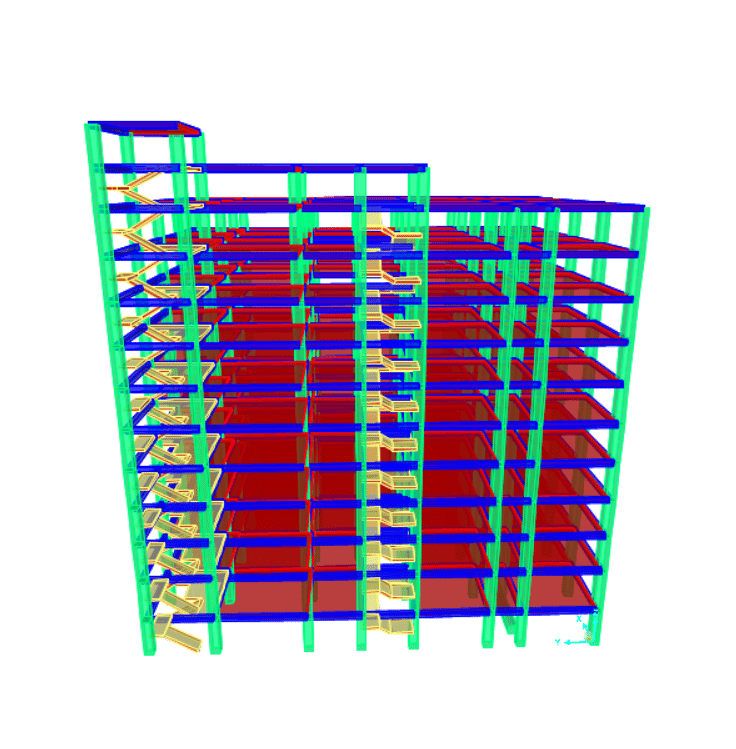
The raft foundation design with step wise procedure are as given:

As per IS 1893: 1 Cl 6.3.5.2 allowable bearing pressure in soil can be increased depending upon type of foundation thus bearing capacity of soil is increased by 50% assuming it will be Raft foundation

Then, q = 1.5×150 = 225 kN/m2 and applying factor of safety of 1.2, q = 187.5 kN/m2

Generally, the depth of raft foundation from ground level shall be not less than 1 m (IS 2950 Part 1, Cl. 4.3)

D_f = 𝑞_𝑢/𝛾_𝑠 × (1−𝑠𝑖𝑛Ø)²/(1+𝑠𝑖𝑛Ø)² = 187.5/18×(1−𝑠𝑖𝑛30)²/(1+𝑠𝑖𝑛30)² = 1.157 m > 1 m OK.

If the value was less than 1 m, the lower face of the designed footing shall have been placed at a level of 1 m below which soil is free from seasonal volumetric change.

The area of foundation required for safe transmission of load, assuming above reaction from superstructure is taken as non-eccentric surcharge to the soil, is given by:

Area of foundation required = Σ𝑃+10% 𝑜𝑓 Σ𝑃𝑞 = 1.1×188190.67 * 187.5 𝑚² = 1104 m²

Plinth area of the building = 1356.78 m²

Hence, % Area of required footing = (1104/1356.78)×100 = 81.37 % > 50%

Consider 500 mm projection for the raft having same shape of the superstructures along the building periphery for critical shear section consideration.

∴ Area of Foundation provided = 1430.97 m²

Location of geometric C.G.: X = 15.24 m, Y = 21.265m

Calculation of Eccentricity:

Column	Service load	X	Y	F*X	F*Y
ID	KN	m	m
A1	3057.298	0	0	0	0
A2	3433.39067	0	7.16	0	24583.08
A3	3546.08333	0	10.32	0	36595.58
A4	4078.51267	0	17.48	0	71292.4
A5	4265.566	0	27.5	0	117303.1
A6	4845.374	0	36.23	0	175547.9
A7	2491.35333	0	42.53	0	105957.3
B1	4537.91333	7.62	0	34578.9	0
B2	5397.60667	7.62	7.16	41129.76	38646.86
B3	5567.01733	7.62	10.32	42420.67	57451.62
B4	6836.08933	7.62	17.48	52091	119494.8
B5	6945.63733	7.62	27.5	52925.76	191005
B6	7042.53333	7.62	36.23	53664.1	255151
B7	4210.06733	7.62	42.53	32080.71	179054.2
C1	4673.90933	15.24	0	71230.38	0
C2	5609.96333	15.24	7.16	85495.84	40167.34
C3	5733.08333	15.24	10.32	87372.19	59165.42
C4	6737.16867	15.24	17.48	102674.5	117765.7
C5	7416.846	15.24	27.5	113032.7	203963.3
C6	7247.21133	15.24	36.23	110447.5	262566.5
C7	4579.06267	15.24	42.53	69784.92	194747.5
D1	4547.16333	22.86	0	103948.2	0
D2	5485.98067	22.86	7.16	125409.5	39279.62
D3	5611.94533	22.86	10.32	128289.1	57915.28
D4	7946.608	22.86	17.48	181659.5	138906.7
D5	8692.12533	22.86	27.5	198702	239033.4
D6	7090.10933	22.86	36.23	162079.9	256874.7
D7	4454.27467	22.86	42.53	101824.7	189440.3
E1	3118.13467	30.48	0	95040.74	0
E2	3641.17867	30.48	7.16	110983.1	26070.84
E3	3718.392	30.48	10.32	113336.6	38373.81
E4	5271.26467	30.48	17.48	160668.1	92141.71
E5	5760.85733	30.48	27.5	175590.9	158423.6
E6	4714.16267	30.48	36.23	143687.7	170794.1
E7	3115.51933	30.48	42.53	94961.03	132503
A8	2641.38267	0	22.49	0	59404.7
Total	184060.786			2845110	3849620

The total service load from the table and initially taken is different because the load of staircase and shear wall should also be taken for the area calculation.

X̅ = (Σ𝐹𝑖×𝑋𝑖)/Σ𝑃 = 15.457 m

Ȳ= (Σ𝐹𝑖×𝑌𝑖)/Σ𝑃 = 20.915 m

Load Centroid (X, Y) = (15.457m, 20.915m)

e_x = 15.457 – 15.24 = 0.217 m

e_y = 21.265 – 20.915 = 0.35 m

M_x = P * e_y = 188190.67 * 0.35 = 65866.74 KN-m

M_y = P * e_x  = 188190.67 * 0.207 = 40837.37 KN-m

I_xx = BD³/12 = 32.28 * 44.33³ /12 = 234340 m⁴

I_yy = DB³/12 = 32.28³ * 44.33 / 12 = 124256 m⁴

Soil Pressure at different Points (corner and edge) are as follows:

Column ID	P/A	Ixx	Iyy	X	Y	Mxx*Y /Ixx	Myy * X/Iyy	Soil pressure
Corner A1	131.5	234340	124256	-16.14	-22.165	-6.230	-5.304	119.966
A2	131.5	234340	124256	-16.14	-14.105	-3.965	-5.304	122.231
A3	131.5	234340	124256	-16.14	-10.945	-3.076	-5.304	123.119
A4	131.5	234340	124256	-16.14	-3.785	-1.064	-5.304	125.132
A5	131.5	234340	124256	-16.14	6.235	1.752	-5.304	127.948
A6	131.5	234340	124256	-16.14	14.965	4.206	-5.304	130.402
Corner A7	131.5	234340	124256	-16.14	22.165	6.230	-5.304	132.425
Corner E1	131.5	234340	124256	16.14	-22.165	-6.230	5.304	130.575
E2	131.5	234340	124256	16.14	-14.105	-3.965	5.304	132.840
E3	131.5	234340	124256	16.14	-10.945	-3.076	5.304	133.728
E4	131.5	234340	124256	16.14	-3.785	-1.064	5.304	135.741
E5	131.5	234340	124256	16.14	6.235	1.752	5.304	138.557
E6	131.5	234340	124256	16.14	14.965	4.206	5.304	141.011
Corner E7	131.5	234340	124256	16.14	22.165	6.230	5.304	143.034
B1	131.5	234340	124256	-7.62	-22.165	-6.230	-2.504	122.766
C1	131.5	234340	124256	0	-22.165	-6.230	0.000	125.270
D1	131.5	234340	124256	7.62	-22.165	-6.230	2.504	127.774
B7	131.5	234340	124256	-7.62	22.165	6.230	-2.504	135.226
C7	131.5	234340	124256	0	22.165	6.230	0.000	137.730
D7	131.5	234340	124256	7.62	22.165	6.230	2.504	140.234

Hence maximum downward stress (140.234 kN/m²) is less than safe bearing capacity (187.5 kN/m2) so OK.

In X-direction raft is divided in seven strips that is into 5 equivalent beam, the beam with the respective soil pressure and moment are as follows.

Bending moment is obtained by coefficient (1/12) and ‘L’ as center to center distance, from IS 456 Cl. 22.5.1

+M = -M = wl²/12

Beams	Strip width	Length L	Coeff.	Soil pressure	Equivalent soil pressure	Soil pressure per strip KN/m2
A-A	4.71	10.02	1/12	132.425	132.425	1107.960
B-B	7.62	10.02	1/12	135.225	133.825	1119.674
C-C	7.62	10.02	1/12	137.73	136.4775	1141.866
D-D	7.62	10.02	1/12	140.23	138.98	1162.804
E-E	4.71	10.02	1/12	143.03	141.63	1184.976
					Maximum	1184.970

In the Y-direction the raft is divided into 7 strips i.e. into seven equivalent beams.

Beams	Strip width	Length L	Coeff.	Soil pressure	Equivalent soil pressure	Soil pressure per strip KN/m2
1	4.48	7.62	1/12	130.57	130.57	631.79
2	5.16	7.62	1/12	132.84	131.71	637.28
3	5.16	7.62	1/12	133.73	133.28	644.92
4	8.59	7.62	1/12	135.74	134.73	651.94
5	9.375	7.62	1/12	138.56	137.15	663.62
6	7.52	7.62	1/12	141.01	139.78	676.37
7	4.05	7.62	1/12	143.03	142.02	687.20
					maximum	687 .20

Therefore, maximum moment is 1184.97 kNm/m per strip.

Calculation of Depth of Foundation:

i. Calculation of Depth from Moment Criterion (IS 456 : 2000, ANNEX G 1.1):

M_u = 0.133 f_ck b d² [for fe500]

1184.97 * 10⁶ = 0.133 * 30 * 1000 * d²

d = 545 mm

ii. Calculation of Depth from Two Way Shear:

Depth of raft will govern by two-way shear at one of the exterior column. In case, location of critical shear is not obvious it may be necessary to check all locations. When shear reinforcement is not provided, the calculated shear stress at critical section shall not exceed K_s×τ_c. i.e. τ_v ≤ K_s×τ_c. (IS 456 : 2000, Cl. 31.6.3.1)

Where,

K_s = (0.5 + β_c) but not greater than 1, βc being the ration of short side to long side of the column/capital; and

τ_c = 0.25 √𝑓_𝑐𝑘 in limit state method of design.

Here, βc = 1

Ks = 1+0.5 = 1.5 > 1

Hence, Ks = 1

Shear strength of concrete (τ_c) = 0.25×√30 = 1.37 N/mm2

For column D5

Factored Column Load = 13038.19 kN

Perimeter (p_o) = 4 (d + 800)

The nominal shear stress in flat slabs shall be taken as V/(p_o×d) where V is the shear force due to design, P₀ is the periphery of the critical section and d is the effective depth of the slab. (IS 456 : 2000, Cl. 31.6.2.1)

𝜏_𝑣=𝑉_𝑢/(𝑝_𝑜×𝑑)

1.37 = 13038.19×10³/[4(𝑑+540)×𝑑]

d = 1193.87 mm

For corner column E7

Factored Column Load = 4677.2 kN

Perimeter po = 2×(0.5d + 800 + 500 + 0.5d + 800+500) = 2×(d + 2600)

𝜏𝑣=𝑉𝑢/(𝑝𝑜×𝑑)=4677.2*10³/ 2(𝑑+2600)×𝑑

𝑂𝑟,1.37=4677.2×10³/ [2(𝑑+2600)×𝑑]

Hence d = 543.33 mm

Hence, the thickness of raft foundation design is governed by two-way shear.

Adopt effective Depth (d) = 1200 mm

Adopt effective cover of 65 mm (IS456: 2000, Cl. 26.4.2.2)

Overall Depth = 1200 + 65= 1265 mm

Reinforcement calculation:

In shorter Direction

We have from (IS 456 : 2000, Annex G 1.1)

BM = 0.87×f_y×A_st×(d – 𝑓_𝑦×𝐴_𝑠𝑡/𝑓𝑐𝑘×𝑏)

Or, 687×10⁶ = 0.87×500×A_st×(1200 – 500×𝐴_𝑠𝑡/ 30×1000)

Solving we get,

Ast = 1341.07 mm2

The mild steel reinforcement in either direction in slab shall not be less than 0.15% of the total cross-sectional area. However this value can be reduced to 0.12% when high strength deformed bar or welded wire fabric are used. (IS 456 : 2000, Cl. 26.5.2.1)

Minimum reinforcement in slab = 0.12%×1000×1265 = 1518 mm²

i.e. A_{st, required} < A_{st, Min.}

So, A_{st, required} = 1518 mm²

Using 16 mm Ø bars,

Spacing of Bar (Sv) = 𝐴_𝑏/𝐴_𝑠𝑡×1000=201.062/1518×1000=132.45 𝑚𝑚

Hence, provide 16 mm Ø Bars @ 120 mm C/C in Shorter direction.

Therefore, A_{st, Provided} = (201.062/120)×1000 = 1675.52 mm²

In Longer Direction:

Adopt effective depth = 1200 – 16 = 1184 mm

Reinforcement in longer direction is given by:  

BM = 0.87×f_y×A_st×(d – 𝑓𝑦×𝐴_𝑠𝑡/𝑓_𝑐𝑘×𝑏)

Or, 1184.97×10⁶ = 0.87×500×A_st×(1184 – 500×𝐴𝑠𝑡/30×1000)

Solving we get A_st = 2380.50 mm²

Minimum reinforcement in slab = 0.12%×1265×1000 = 1518 mm²

i.e. A_{st required} > A_stMin. OK.

Using 16 mm Ø bars,

Spacing of Bar (S_v) = 𝐴_𝑏/𝐴_𝑠𝑡×1000=201.062/2380.50×1000= 84.46 𝑚𝑚

Hence, Provide 16 mm Ø @ 80 mm C/C in Longer direction.

Therefore, Ast provided = (201.062/80)×1000 = 2513.275 mm2

Check for Development Length:

Bond Stress (𝜏_𝑏𝑑)=1.4 𝑁/𝑚𝑚2, For M30 Concrete. This value can be increased by 60% for Tor Steel. (IS 456 : 2000, Cl. 26.2.1.1)

The development length (Ld) is given by (IS 456 : 2000, Cl. 26.2.1)

𝐿_𝑑=∅×𝜎_𝑠/4×𝜏_𝑏𝑑= ∅×0.87×500/(4×1.6×1.6)=42.48∅

L_d = 42.48×16 = 679.68 mm

𝐿_𝑑≤ 1.3×𝑀1/𝑉+𝑙_𝑜 (IS 456 : 2000, Cl. 26.2.1)

l_o = Effective depth or 12∅, Whichever is greater.

𝐿𝑑≤ 1.3×1184.97×10⁶ /4677.2×1000+1200=1529.08 𝑚𝑚 OK.

Load Transfer from Column to footing:

Nominal bearing stress in column concrete (σ_br) = P_u/A_c

= (13038.19×1000)/(800×800) = 20.37 N/mm²

Allowable bearing stress = 0.45×fck = 0.45×30 = 13.5 N/mm2 (IS 456: 2000, Cl. 34.4)

If the permissible bearing stress on the concrete within the supporting or supported members exceeded, reinforcement shall be provided for developed surplus force by dowels. (IS 456: 2000, Cl. 34.4.1)

Dowel of at least 0.5% of the cross-sectional area of the supported column and a minimum of four bars shall be provided. Diameter of the Dowels shall not exceed the diameter of column bar by more than 3 mm. (IS 456 : 2000, Cl. 34.4.1)

Area of Dowels Bar = 0.5%×800×800 = 3200 mm²

Provide 28 mm ∅ as Dowel bar.

Development length for dowel bar = ∅×𝜎_𝑠/4×𝜏_𝑏𝑑= 28×0.87×500/4×1.6×1.6=1189.45 𝑚𝑚

Use 6 – 28 mm ∅ Bars as Dowel Bar, then As, provided = 6×PI×28²/4 = 3694.5 mm² > 3200 mm²

Also read:

Design of isolated footing